Baker's percentage formula can be a somewhat confusing because you do not end up at 100% when you add all the percentages together. You also have to use a bit of math to figure out how to mix your own breads. But it has some major advantages.

• You can easily convert a recipe if you need to make 25 bread instead of 20
• It's easier to remember recipes as a percentage formula since they are the same regardless of the amount of bread and the size of the loaf.
• You'll get easier overview of "patterns" in your recipes so you understand bread better. You will get an instinctive feel for any bread recipe.
• You can easily change a small part of the recipe in an educated manner and see what effect it has. 

In the examples below I use the metric system. If you find that confusing then just think of gram (g) as ounce (oz). The important thing is that you just use one unit. So no mixing of oz and lbs for example. Then the calculations will be all the same.

How does it work?

You calculate any ingredients "percentage" relative to the total amount of flour in the recipe. This includes whole flour, flour kernels, whole wheat, rye flour, etc etc. Any flour whatsoever and you add them together and find the total weight.

This weight is defined as "100%". The rest of the ingredients of the recipe are then calculated in relation to that total weight.

A normal recipe (example) - step 1

A simple recipe for spelt bread can be:

• 375 g of wheat flour
• 125 g spelt flour
• 2 tsp salt
• 4 dl water
• yeast the size of a pea

The above recipe use a mix of weight and volume: gram, dl (100 ml), tsp, pea. This is perfectly normal, and is often used to make the measurements faster in a home kitchen. But it is unusable for calculating the bakers percentage.

First, we need to convert all the ingredient values into weight - Step 2

• 375 g of wheat flour
• 125 g spelt flour
• 14 g of salt (1 teaspoon = 7 grams) 
• 400 g of water
• 2 g of yeast

The total flour weight (tfw) - Step 3

tfw= 375 g wheat flour + 125 g spelt flour = 500 g total flour

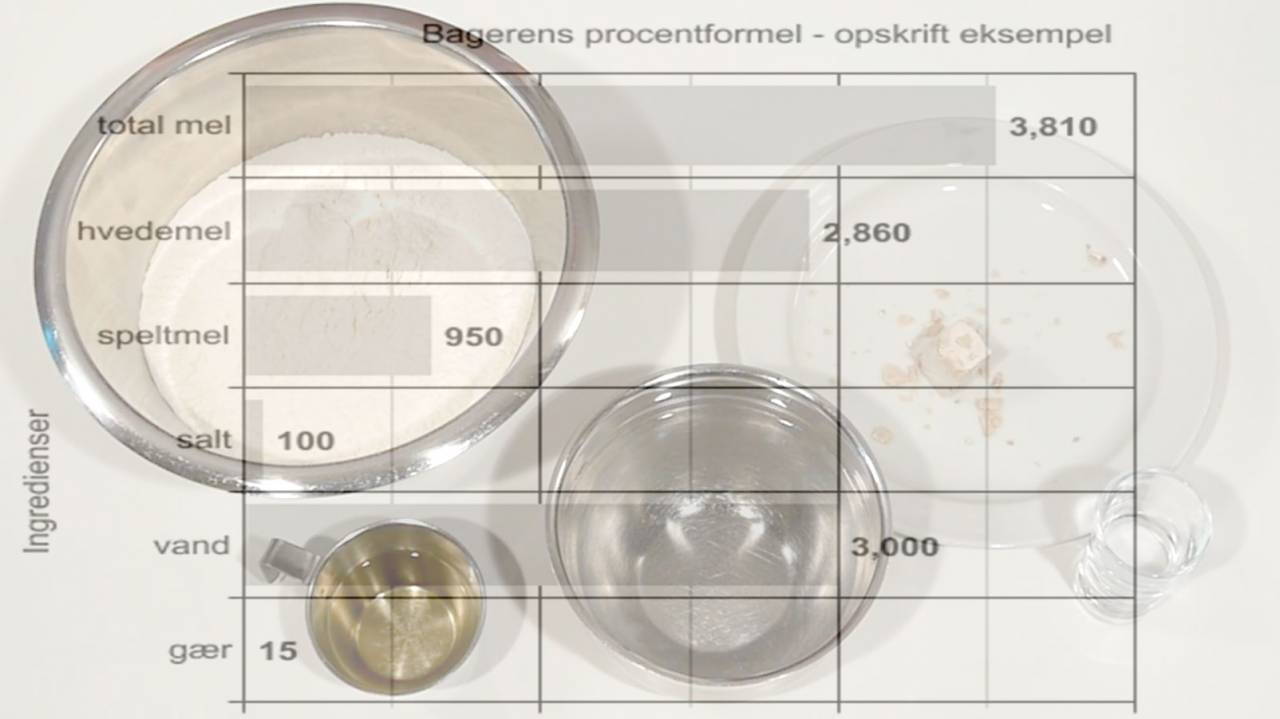
At this stage we can compare the amounts directly. We can even put them in a graph. You don't usually do this, but it might visualise the principle better. So here goes.

The ingredient percentage from their weight - step 4

Now that we got comparable weights we can use those to calculate the percentages.

To find the ingredients percent use the formula:

Ingredient %: (ingredient weight / tfw) * 100

total flour %: 100% - per definition.

flour %: (375 g / 500 g) * 100 = 75% wheat flour compared to total flour

spelt flour %: (125 g / 500 g) * 100 = 25% spelt flour (I usually don't use more than 25% non-wheat flour in my recipes)

salt %: (14 g / 500 g) * 100 = 2.8% salt (bread typically has a salt content of 1.5% - 2.5% salt, so this is a little more salty than usual.)

water %: (400 g / 500 g) x 100 = 80% water (this is a wet dough)

yeast %: (2 g / 500 g) * 100 = 0.4% yeast (This small amount of yeast suggests a long proving time.)

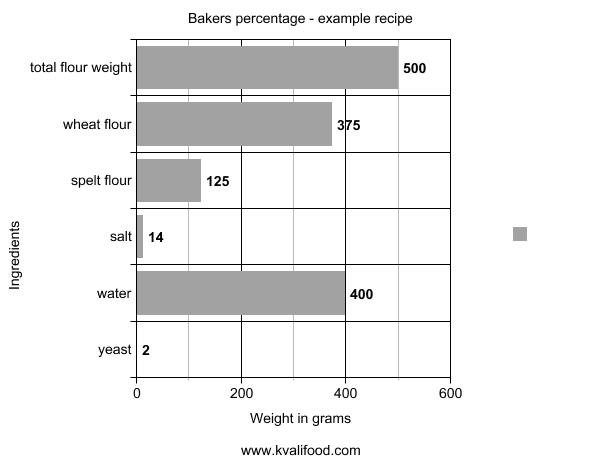
The recipe expressed in baker's percentage - Step 5

• 75% wheat flour
• 25% spelt flour
• 2.8% salt
• 80% water
• 0.4% yeast

The total percentage (tp) - Step 6

This is the part that makes the lest sense in bakers percentage. That the total percentage is not 100% as one would assume when adding all ingredients in a recipe. But this way of doing it has the advantage that it is easier to compare recipes.

So to add it up: total percentage (tp) = 75 +25 +2.8 +80 +0.4 = 183.2%

The total weight (tw) - Step 7

When knowing total percentage (tp)  and the total flour weight (tfw) is possible to calculate the total weight of the bread by the formula:

tw = (tp * tfw) / 100 = (500 * 183.2) / 100 = 916 g or approx. 1 kg. (2 lbs) which is a very normal weight for a large loaf of bread.

Conversion to other volumes

An advantage of the bakers percentage is that it is easy to calculate new weights for other amounts of bread. We just need to find out how much weight each percentage point each corresponds to, and then multiply with that.

If we need 10 loaves of 700 g we can get the recipe in grams like this:

10 bread * 700 grams = 7000 g

In metric values:

7000 g / 183.2% = 38.2 g/%. What this number says it that each percentage point in the recipe corresponds to 38.2 g.

Or in empirical values (7000g = 246 oz):

246 oz  / 183.2% = 1.34 oz/% each percentage point in the recipe corresponds to 1.34 oz.

All ingredients are calculated like this - step 8:

Then we just need to multiply all the ingredients with the weight per percentage:

wheat flour: 38.2 * 75 = 2,865 g

spelt flour: 38.2 * 25 = 955 g

salt: 38.2 * 2.8 = 107 g

water: 38.2 * 80 = 3,056 g

yeast: 38.2 * 0.4 = 15.3 g

These numbers has a precision that is not needed and looks confusing. So the recipe is hard to read, and it is implied that it is important that you use 2,865 g flour and not 2,866 g. Which makes absolutely no difference in practice. To get a nicer recipe we round them up.

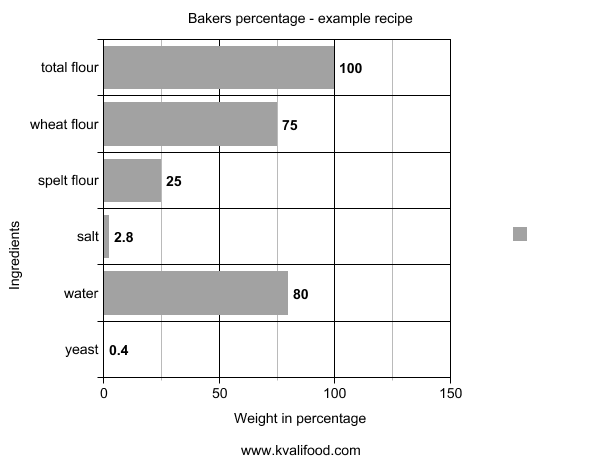
For 10 loaves of 700 g each the recipe by weight is then - step 9:

• 2,860 g wheat flour
• 950 g spelt flour
• 100 g of salt
• 3000 g (3 liters) of water
• 15 g of yeast

Which is the final recipe. And as you can see in the  graph below, the relative values are exactly the same in the final recipe as in the first one. Only there is more of each thing.  Which is as it should be.

Regarding starters / pre-ferments

A starter is a small amount of dough or batter (like a sourdough) that you make in advance and then use instead of yeast.

This complicates matters a bit, as a starter also contains flour and liquid. Should they be counted as part of the %'es?

It is valid to see the starter as a separate ingredient. So you just calculate that as a percentage too.

This is the way I usually do it.

• pro: easy to use for pre ferments that you can just make any amount of in any hydration you prefer.
• con: Does not represent the true value of flour and liquid in the bread.

But it is also valid to calculate the values of the ingredients in the starter as part of the total amounts. So that the flour in the starter + the flour in the recipe = 100%

• pro: gives the correct values for all the ingredients.
• con: you must make a starter with a precise hydration and in a precise weight. Or take the ingredients of the starter from the recipes ingredients.

sourdough

sourdough